
Spherical Coordinates To Cylindrical Coordinates How To Convert From
The cylindrical (left) and spherical (right) coordinates of a Cylindrical coordinates is a method of describing location in a three-dimensional coordinate system. Triangles and trigonometry prove to be particularly important. Our goal is to consider some examples of how to convert from rectangular coordinates to each of these systems, and vice versa. In the following questions, we investigate the two new coordinate systems that are the subject of this section: cylindrical and spherical coordinates.
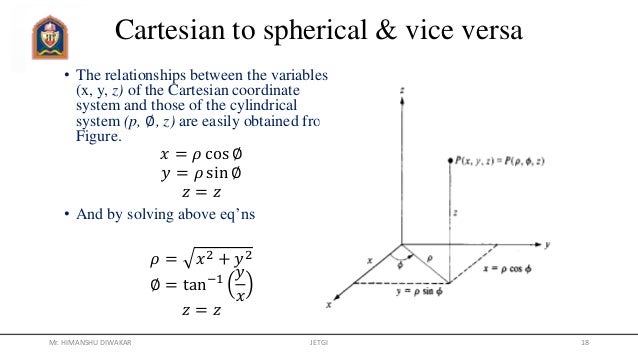
(r, ) are the polar coordinates of the point’s projection in the xy -plane. In the cylindrical coordinate system, a point in space (Figure 5.7.1) is represented by the ordered triple (r, , z), where. Go through the following article for in depth discussion of the Curl.Definition: The Cylindrical Coordinate System. The uniform vector field posses zero curl. The net circulation may be positive or negative.
Where do they come from? What is the logic behind them. Later by analogy you can work for the spherical coordinate system.As read from above we can easily derive the Curl formula in Cartesian which is as below.And the same in cylindrical coordinates is as follows:It is quite obvious to think that why some extra terms like (1/ρ) and ρ are present. Go through this article for intuitive derivation.Deriving Curl in Cylindrical and SphericalLet’s talk about getting the Curl formula in cylindrical first. Now, consider a Spherical element as shown in the figure.The Curl formula in cartesian coordinate system can be derived from the basic definition of the Curl of a vector field.
...
Check out this related article. The derivatives of the unit vectors is not zero always. Don’t consider unit vector to be a constant and taking out of the derivative.


 0 kommentar(er)
0 kommentar(er)
